Friday, August 10, 2012

Orthogonal and Symplectic Lie Algebras

Orthogonal and Symplectic Lie Algebras:
For the next three families of linear Lie algebras we equip our vector space V with a bilinear form 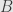B. We’re going to consider the endomorphisms f\in\mathfrak{gl}(V) such that
\displaystyle B(f(x),y)=-B(x,f(y))
If we pick a basis \{e_i\} of V, then we have a matrix for the bilinear form
\displaystyle B_{ij}=B(e_i,e_j)
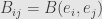
and one for the endomorphism
\displaystyle f(e_i)=\sum\limits_jf_i^je_j
So the condition in terms of matrices in \mathfrak{gl}(n,\mathbb{F}) comes down to
\displaystyle\sum\limits_kB_{kj}f_i^k=-\sum_kf_j^kB_{ik}
or, more abstractly, Bf=-f^TB.
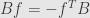
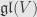
So do these form a subalgebra of \mathfrak{gl}(V)? Linearity is easy; we must check that this condition is closed under the bracket. That is, if f and g both satisfy this condition, what about their commutator [f,g]?
\displaystyle\begin{aligned}B([f,g](x),y)&=B(f(g(x))-g(f(x)),y)\\&=B(f(g(x)),y)-B(g(f(x)),y)\\&=-B(g(x),f(y))+B(f(x),g(y))\\&=B(x,g(f(y)))-B(x,f(g(y)))\\&=-B(x,f(g(y))-g(f(y)))\\&=-B(x,[f,g](y))\end{aligned}
So this condition will always give us a linear Lie algebra.
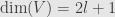
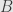
We have three different families of these algebras. First, we consider the case where \mathrm{dim}(V)=2l+1 is odd, and we let B be the symmetric, nondegenerate bilinear form with matrix
\displaystyle\begin{pmatrix}1&0&0\\ 0&0&I_l\\ 0&I_l&0\end{pmatrix}
where I_l is the l\times l identity matrix. If we write the matrix of our endomorphism in a similar form
\displaystyle\begin{pmatrix}a&b_1&b_2\\c_1&m&n\\c_2&p&q\end{pmatrix}
our matrix conditions turn into
\displaystyle\begin{aligned}a&=0\\c_1&=-b_2^T\\c_2&=-b_1^T\\q&=-m^T\\n&=-n^T\\p&=-p^T\end{aligned}
From here it’s straightforward to count out 2l basis elements that satisfy the conditions on the first row and column, \frac{1}{2}(l^2-l) that satisfy the antisymmetry for p, another \frac{1}{2}(l^2-1) that satisfy the antisymmetry for n, and l^2 that satisfy the condition between m and q, for a total of 2l^2+l basis elements. We call this Lie algebra the orthogonal algebra of V, and write \mathfrak{o}(V) or \mathfrak{o}(2l+1,\mathbb{F}). Sometimes we refer to the isomorphism class of this algebra as B_l.
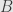
Next up, in the case where \mathrm{dim}(V)=2l is even we let the matrix of B look like
\displaystyle\begin{pmatrix}0&I_l\\I_l&0\end{pmatrix}
A similar approach to that above gives a basis with 2l^2-l elements. We also call this the orthogonal algebra of V, and write \mathfrak{o}(V) or \mathfrak{o}(2l,\mathbb{F}). Sometimes we refer to the isomorphism class of this algebra as D_l.
Finally, we again take an even-dimensional V, but this time we use the skew-symmetric form
\displaystyle\begin{pmatrix}0&I_l\\-I_l&0\end{pmatrix}
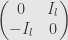
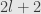
This time we get a basis with 2l+2 elements. We call this the symplectic algebra of V, and write \mathfrak{sp}(V) or \mathfrak{sp}(2l,\mathbb{F}). Sometimes we refer to the isomorphism class of this algebra as C_l.
Along with the special linear Lie algebras, these form the “classical” Lie algebras. It’s a tedious but straightforward exercise to check that for any classical Lie algebra L, each basis element e of L can be written as a bracket of two other elements of L. That is, we have [L,L]=L. 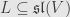Since L\subseteq\mathfrak{gl}(V) for some V, and since we know that [\mathfrak{gl}(V),\mathfrak{gl}(V)]=\mathfrak{sl}(V), this establishes that L\subseteq\mathfrak{sl}(V) for all classical L.



DIGITAL JUICE
Posted by at

No comments:

Post a Comment

Thank's!

Subscribe to: Post Comments (Atom)