The category of Lie algebras may not be Abelian, but it has a zero object, kernels, and cokernels, which is enough to get the first isomorphism theorem, just like for rings. Specifically, if
is any homomorphism of Lie algebras then we can factor it as follows:
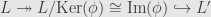
That is, first we project down to the quotient of
by the kernel of 
, then we have an isomorphism from this quotient to the image of , followed by the inclusion of the image as a subalgebra of 
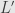
.There are actually two more isomorphism theorems which I haven’t made much mention of, though they hold in other categories as well. Since we’ll have use of them in our study of Lie algebras, we may as well get them down now.
The second isomorphism theorem says that if
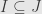
are both ideals of , then 
is an ideal of 
. Further, there is a natural isomorphism 
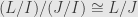
. Indeed, if 
and 
, then we can check that![\displaystyle[x+I,j+I]=[x,j]+I\in J/I](http://s0.wp.com/latex.php?latex=%5Cdisplaystyle%5Bx%2BI%2Cj%2BI%5D%3D%5Bx%2Cj%5D%2BI%5Cin+J%2FI&bg=e6e6e6&fg=333333&s=0 "\displaystyle[x+I,j+I]=[x,j]+I\in J/I")
so
is an ideal of . As for the isomorphism, it’s straightforward from considering 
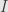
and 
as vector subspaces of . Indeed, saying 
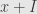
and 
are equivalent modulo in is to say that 
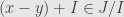
. But this means that 
for some 
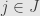
, so 
and 
are equivalent modulo in .The third isomorphism theorem states that if
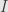
and are any two ideals of , then there is a natural isomorphism between 
and 
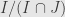
— we showed last time that both 
and 
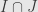
are ideals. To see this, take 
and 
in and consider how they can be equivalent modulo . First off, 
and 
are immediately irrelevant, so we may as well just ask how 
and 
can be equivalent modulo . Well, this will happen if 
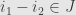
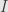
, but we know that their difference is also in , so 
.
DIGITAL JUICE
No comments:
Post a Comment
Thank's!