It turns out that all the derivations on a semisimple Lie algebra
are inner derivations. That is, they’re all of the form 
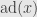
for some 
. We know that the homomorphism 
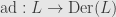
is injective when is semisimple. Indeed, its kernel is exactly the center 
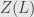
, which we know is trivial. We are asserting that it is also surjective, and thus an isomorphism of Lie algebras.If we set
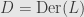
and 
, we can see that ![[D,M=I]\subseteq I](http://s0.wp.com/latex.php?latex=%5BD%2CM%3DI%5D%5Csubseteq+I&bg=e6e6e6&fg=333333&s=0 "[D,M=I]\subseteq I")
. Indeed, if 
is any derivation and , then we can check that&=\delta([\mathrm{ad}(x)](y))-[\mathrm{ad}(x)](\delta(y))\\&=\delta([x,y])-[x,\delta(y)]\\&=[\delta(x),y]+[x,\delta(y)]-[x,\delta(y)]\\&=[\mathrm{ad}(\delta(x))](y)\end{aligned}](http://s0.wp.com/latex.php?latex=%5Cdisplaystyle%5Cbegin%7Baligned%7D%5Cleft%5B%5Cdelta%2C%5Cmathrm%7Bad%7D%28x%29%5Cright%5D%28y%29%26%3D%5Cdelta%28%5B%5Cmathrm%7Bad%7D%28x%29%5D%28y%29%29-%5B%5Cmathrm%7Bad%7D%28x%29%5D%28%5Cdelta%28y%29%29%5C%5C%26%3D%5Cdelta%28%5Bx%2Cy%5D%29-%5Bx%2C%5Cdelta%28y%29%5D%5C%5C%26%3D%5B%5Cdelta%28x%29%2Cy%5D%2B%5Bx%2C%5Cdelta%28y%29%5D-%5Bx%2C%5Cdelta%28y%29%5D%5C%5C%26%3D%5B%5Cmathrm%7Bad%7D%28%5Cdelta%28x%29%29%5D%28y%29%5Cend%7Baligned%7D&bg=e6e6e6&fg=333333&s=0 "\displaystyle\begin{aligned}\left[\delta,\mathrm{ad}(x)\right](y)&=\delta([\mathrm{ad}(x)](y))-[\mathrm{ad}(x)](\delta(y))\\&=\delta([x,y])-[x,\delta(y)]\\&=[\delta(x),y]+[x,\delta(y)]-[x,\delta(y)]\\&=[\mathrm{ad}(\delta(x))](y)\end{aligned}")
This makes
an ideal, so the Killing form 
of 
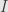
is the restriction of 
of the Killing form of 
. Then we can define 
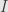
to be the subspace orthogonal (with respect to ) to , and the fact that the Killing form is nondegenerate tells us that 
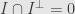
, and thus ![[I,I^\perp]=0](http://s0.wp.com/latex.php?latex=%5BI%2CI%5E%5Cperp%5D%3D0&bg=e6e6e6&fg=333333&s=0 "[I,I^\perp]=0")
.Now, if
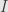
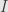
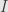
is an outer derivation — one not in — we can assume that it is orthogonal to , since otherwise we just have to use to project onto and subtract off that much to get another outer derivation that is orthogonal. But then we find that![\displaystyle\mathrm{ad}(\delta(x))=[\delta,\mathrm{ad}(x)]=0](http://s0.wp.com/latex.php?latex=%5Cdisplaystyle%5Cmathrm%7Bad%7D%28%5Cdelta%28x%29%29%3D%5B%5Cdelta%2C%5Cmathrm%7Bad%7D%28x%29%5D%3D0&bg=e6e6e6&fg=333333&s=0 "\displaystyle\mathrm{ad}(\delta(x))=[\delta,\mathrm{ad}(x)]=0")
since this bracket is contained in
![[I^\perp,I]=0](http://s0.wp.com/latex.php?latex=%5BI%5E%5Cperp%2CI%5D%3D0&bg=e6e6e6&fg=333333&s=0 "[I^\perp,I]=0")
. But the fact that 
is injective means that 
for all , and thus 
. We conclude that 
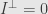
and that 
, and thus that is onto, as asserted.
DIGITAL JUICE
No comments:
Post a Comment
Thank's!